![189079_553855716620_2137_n[1]](https://www.renaissancemusings.com/wp-content/uploads/2013/10/189079_553855716620_2137_n1.jpg)
The most inadvertently sexy math problem in existence, if I may say so myself. [link removed](Don’t worry, it wasn’t explicit, just low-quality).
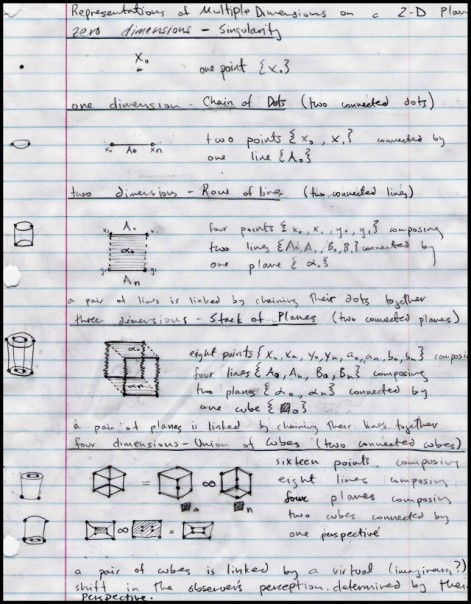
If you’re interested in what went into the thinking process behind the continued exploration of these drawings, the work was initially intended as a study of simple dimentional analysis, before moving on to tackle the symmetrical aesthetics of organisms. It makes a difference if the number of nodes in the tessellated shapes are even, or odd, or prime when paired with the base in which counting is taking place in a counting organisms’ physiology. The project was brought about by infatuation with simple marine life and the maximum complexity of limbs of soft-formed organisms ending with octopi and squid beneath the ocean’s surface, while keeping in mind that most life has a choice between feeding and producing offspring in famine environments. As each organ on a creature’s physical body is paired with a concentration of nerves and impulses, I thought it would be interesting to approximate these as nodes in simple life forms and see what emerged.
The fundamental idea of my additional work is that you may take a single geometric shape (in this case a square) and increase and decrease it’s dimentional footprint to study it’s appearance. Additionally, you may increase or decrease the number of verteces on the shape in order to study the forms of geometrical shapes with different appearances, such as triangles and octagons. While they may appear indistinguishable from points and lines and planes and solids in small dimentions, I’ve begun to start believing that points are not the whole story and that even zero-dimentional objects hold a property of having higher-order verteces, which are manifested when they are represented in higher dimentions. I’ve begun a second drawing to explain, and will post it when it’s finished.
I’m unabashedly proud of the potential for advancement of geometrical modeling for polygonal computer images that rely on n-gons instead of 3-gon pixels with my new work. This is expandable all the way up to infinity, if the limit of sides is taken to the next logical node, one step at a time, from where the work leaves off. In terms of available mathematical computability and linear progression of increasingly infinite nested geometries, the only way to limit to the user’s ability to draw vertices on a given model is to limit the available processing power.
There are also possible applications in plastic surgery, for prosthetics and cosmetic surgeries, as well as for informing physical therapy guidelines. This could also be used for spherical approximation of pi, astrophysics and particle physics modeling, and visual simulations of multiple forms. It could also be considered important for post-modernist technological fabrication using home-based 3D printers, geological cartography, zero-gravity extra-planetary cloud satellite navigation, and self-similar facility construction with ordered and central nodes. I’m sorry for bringing to light a few negative ideas, if that seems a like a bit of a stretch. It’s never a good idea to discount a possibility until it has been explored thoroughly.


Leave a Reply
You must be logged in to post a comment.